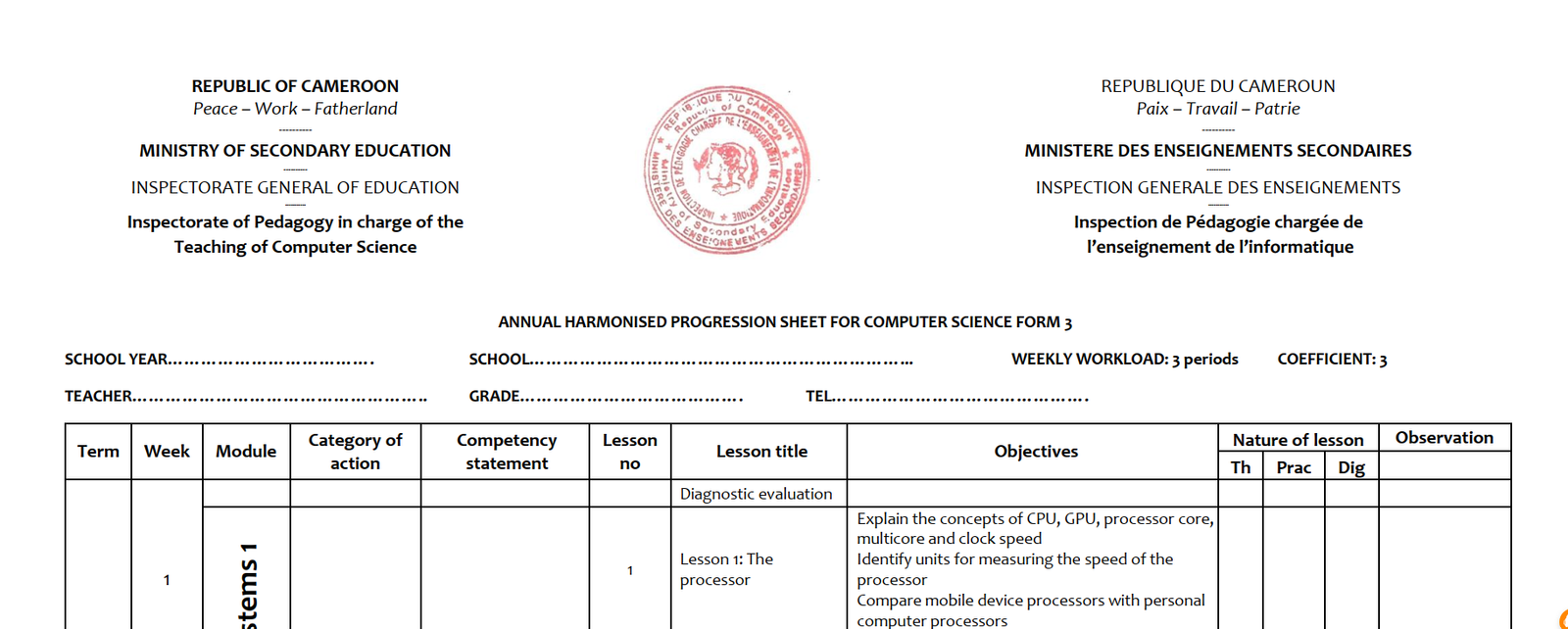
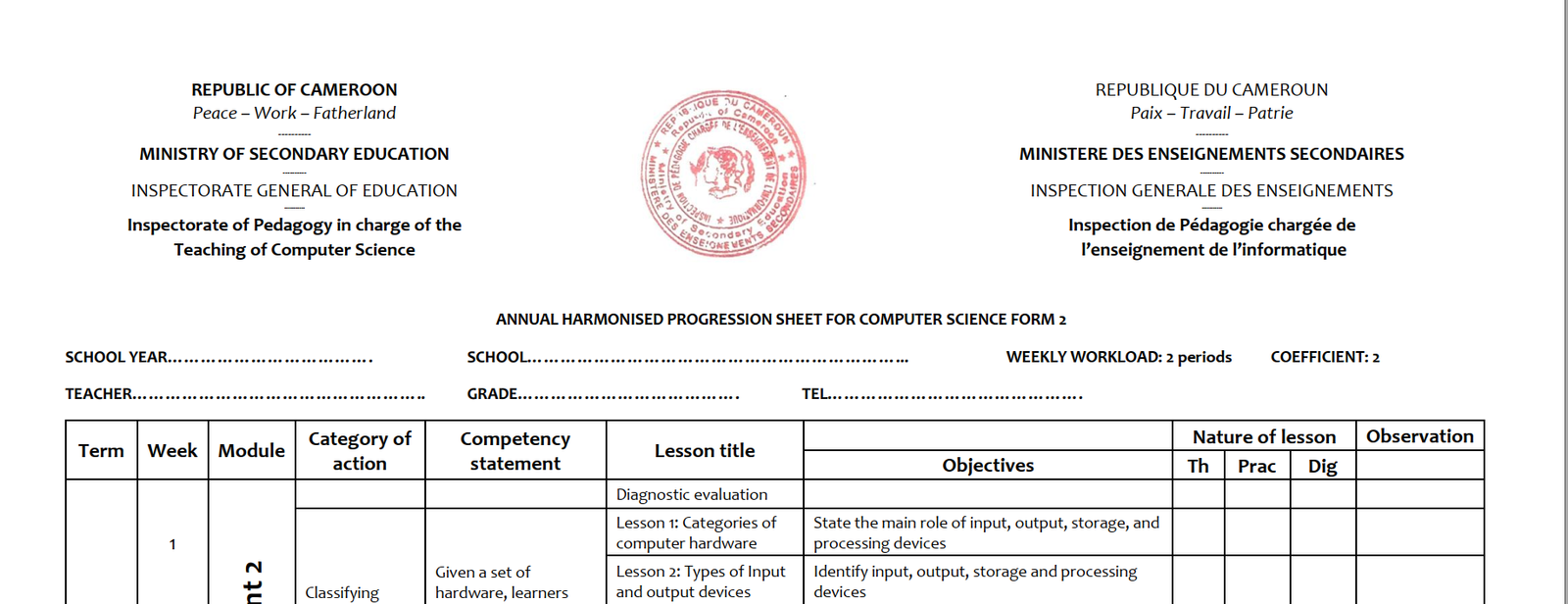
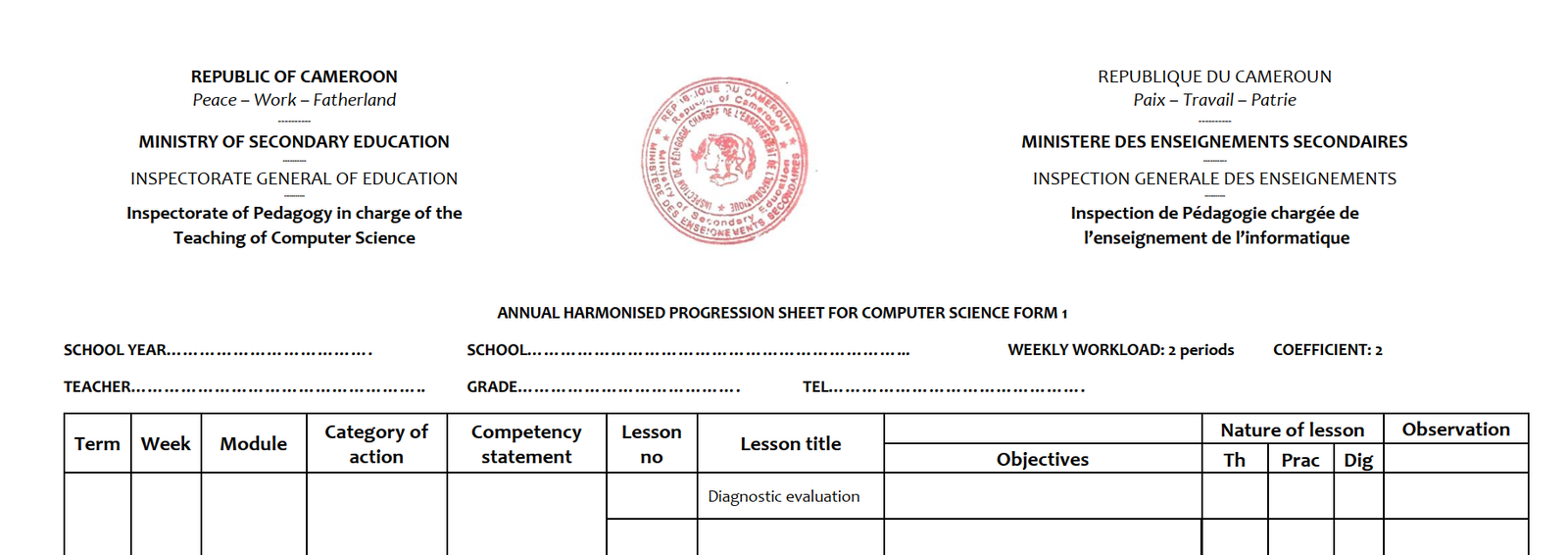
cameroon gce A level June 2024 pure mathematics with mechanics 3
cameroon gce A level June 2024 pure mathematics with mechanics 3
The position vector r of a particle at time t is given by
r = f(2t + 3)i+ (t2 -l)j] m.
(5 marks)’
(6 marks)
(2 marks)
Find the
(a) average velocity over the interval t = 0 to t = 3 s,
(b) speed and distance of the particle from )hc origin when.
(c) Cartesian equation of its path.
J T
2. A ear travels on a straight horizontal track. The car decelerates uniformly from a speed
of 20 m s-l to a speed of 12 m s-l as it travels a distance of 640 m. The car then
(accelerate a) tiiiTe that ^ iinjlor it takes ^ilythe , travelling car to traycl a further the first 1820 640 m in in,70 s. Calculate the
(b) deceleration of the car ciiiring’the -first -640 m,
(c) acceleration ofthc.car.as it travels the further 1820 m,
(d) ispeed of the car when it has completed the further 1820
(e) average speed of the car as it travels the 2460 m.
3. Two Particles P and Q, of masses 6 kg and 4 kg respectively, are connected by a light
incxtensible string of length 2 m. The string passes over a light smooth pulley fixed at the
top ol a smooth planewhich is inclined at an angle a to the horizontal, where sin tt = –
Initially, the particles are held at rest with the string taut, P lying on the plane and Q
hanging over the pulley. The particles are then released from rest.
(a) Find the magnitude of the acceleration of the particles and the tension in the string.
(6 marks)
Given that particle Q is initially 1.5 m above the ground, and particle P does not reach the
top of the Plane. Calculate the
(b) speed which particle Q hits the ground,
(c) time that elapses between when Q reaches the ground and when the string becomes
taut again.
(3 marks)
(4 marks)
( Take g as 10 m s’2.)
4. Particles A and B ,of masses 6 kg and 2 kg respectively, collide directly. The speeds of
A and B after collision are 4 ms-1 and 6 ms”1 respectively and the coefficient of
restitution between A and B is-.
3
(a) Calculate the initial velocities of A and B given that they were moving in the same
/
B moves and hits directly another stationary particle C of mass m kg, causing C to gain
a speed of 10 m sT1. Find
(b) m,
(c) the kinetic energy lost by the system.
(4 marks)
(3 marks)
5. (i) A uniform ladder AB, of weight 40 N and length 8 m, is resting with A against a
smooth vertical wall and B on rough horizontal ground. The coefficient of friction
between the ladder and the ground is The ladder makes an angle 6 with the horizontal
3
ground, where tan 9 = -. Given that, towards the wall, a horizontal force of magnitude
125 N is applied at a point D on the ladder, where BD = 1.5 m, find how far
up the ladder a man of weight 200 N will climb before the ladder will begin to slip.
(it) An elastic string AB of natural length 1.5 m is hanging from a fixed point A. A
of 5 kg is suspended from the end B and produces an extension of 3 mm. calculate the
(a) modulus of elasticity of the string,
(b) elastic potential energy of the string.
( Take g as 10 m s’2)
(8 marks)
mass
(2 marks)
(3 marks)